Is There A Pattern For Prime Numbers
Is There A Pattern For Prime Numbers - This highlights how despite not being discovered yet there must be a solution to how they slot in. Web one interesting aspect of the binary representation of prime numbers is that there are a few patterns, take this code for example at the bottom. For example, the first 5 prime numbers are 2, 3, 5, 7, and 11. It turned out that there is a certain pattern in the placement. Once, during a boring report, stanislav ulam had. Final digit in consecutive numbers is not as random as expected. It can be observed more clearly on a. Web $\begingroup$ certainly there are simple algorithms that will allow you to produce prime after prime by simply proceeding through the natural numbers one by one, but the problem with these algorithms is that at each successive prime takes longer and longer to find, so in the end it is an unfeasible method for characterizing the primes. 1 and 1), is the sum of the previous 2 numbers. But this is impossible since there is no prime that divides 1 and as a result \(q\) is not one of the primes listed. For example, the first 5 prime numbers are 2, 3, 5, 7, and 11. This is true for all values of x. Then f(n) 6 0 mod p, so (iv) holds. The following theorem explains the gaps between prime numbers: Once, during a boring report, stanislav ulam had. Web but there’s no discernable pattern in the occurrence of the primes. This is true for all values of x. Web $\begingroup$ the question in the title, has anyone found a pattern in prime numbers, the answer depends on what you call a pattern. 1, 7, 11, 13, 17, 19, 23, 29 1, 7, 11, 13, 17, 19, 23, 29.. Web now, however, kannan soundararajan and robert lemke oliver of stanford university in the us have discovered that when it comes to the last digit of prime numbers, there is a kind of pattern. This highlights how despite not being discovered yet there must be a solution to how they slot in. The first prime number is 2, and it’s. Web is there a pattern behind prime numbers? Rather all primes are of that form. It turned out that there is a certain pattern in the placement. 1 and 1), is the sum of the previous 2 numbers. Web just think about it: Web this was the first big step towards solving a major question in number theory—whether there are infinitely many pairs of primes that differ by just 2 units, such as the primes 5 and 7 or 11 and 13. Prime time prime numbers are loathe to repeat the final digit of the prime that. The other question you ask, whether. It can be observed more clearly on a. Once, during a boring report, stanislav ulam had. It turned out that there is a certain pattern in the placement. As we can clearly see, there is a definite pattern. Web this was the first big step towards solving a major question in number theory—whether there are infinitely many pairs of primes. Web is there a pattern behind prime numbers? Web $\begingroup$ certainly there are simple algorithms that will allow you to produce prime after prime by simply proceeding through the natural numbers one by one, but the problem with these algorithms is that at each successive prime takes longer and longer to find, so in the end it is an unfeasible. If x is a prime number, the result is an integer multiple of π; Web mathematicians find a peculiar pattern in primes. The other question you ask, whether anyone has done the calculations you have done, i'm sure the answer is yes. Final digit in consecutive numbers is not as random as expected. Once, during a boring report, stanislav ulam. Final digit in consecutive numbers is not as random as expected. The other question you ask, whether anyone has done the calculations you have done, i'm sure the answer is yes. It can be observed more clearly on a. Web this was the first big step towards solving a major question in number theory—whether there are infinitely many pairs of. 1 and 1), is the sum of the previous 2 numbers. Web already there’s a pattern here. The following theorem explains the gaps between prime numbers: Web prime numbers are numbers that have only 2 factors: As we can clearly see, there is a definite pattern. We've been studying primes for a long time, and no one spotted this. Beyond the obvious — after the numbers 2 and 5, primes can’t be even or end in 5 — there seems to be little structure. If x is a prime number, the result is an integer multiple of π; Web each of those four cases is divisible by 2 or 3. Web just think about it: The first prime number is 2, and it’s the only even number since it divides all other even numbers. Once, during a boring report, stanislav ulam had. Fun drawing natural numbers in a spiral and circling only prime. For example, let us consider the following sequences of numbers: Every true (which is odd) is followed by a number that ends with the same number. For example, this prime 13, is an odd binary number, followed by 3 numbers that end with 0 before the next prime. Web now, however, kannan soundararajan and robert lemke oliver of stanford university in the us have discovered that when it comes to the last digit of prime numbers, there is a kind of pattern. Web $\begingroup$ the question in the title, has anyone found a pattern in prime numbers, the answer depends on what you call a pattern. But this is impossible since there is no prime that divides 1 and as a result \(q\) is not one of the primes listed. Web $\begingroup$ certainly there are simple algorithms that will allow you to produce prime after prime by simply proceeding through the natural numbers one by one, but the problem with these algorithms is that at each successive prime takes longer and longer to find, so in the end it is an unfeasible method for characterizing the primes. 1 and 1), is the sum of the previous 2 numbers.
Gann's Square of Nine and Spiral Properties of Prime numbers

Prime Numbers Definition, Prime Numbers 1 to 100, Examples

A Pattern in Prime Numbers ? YouTube
Prime Number Patterns

Prime Numbers Definition, Prime Numbers 1 to 100, Examples
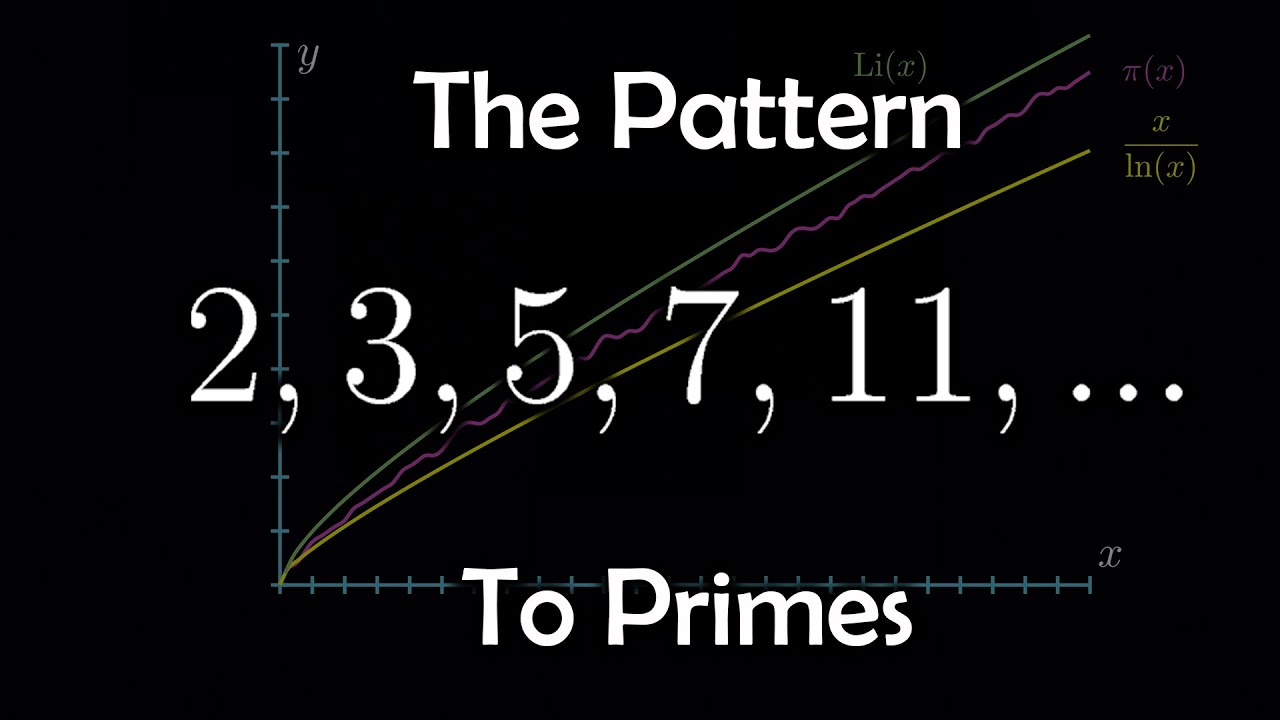
The Pattern to Prime Numbers? YouTube

Plotting Prime Numbers Jake Tae

Prime Number Patterning! The Teacher Studio Learning, Thinking, Creating
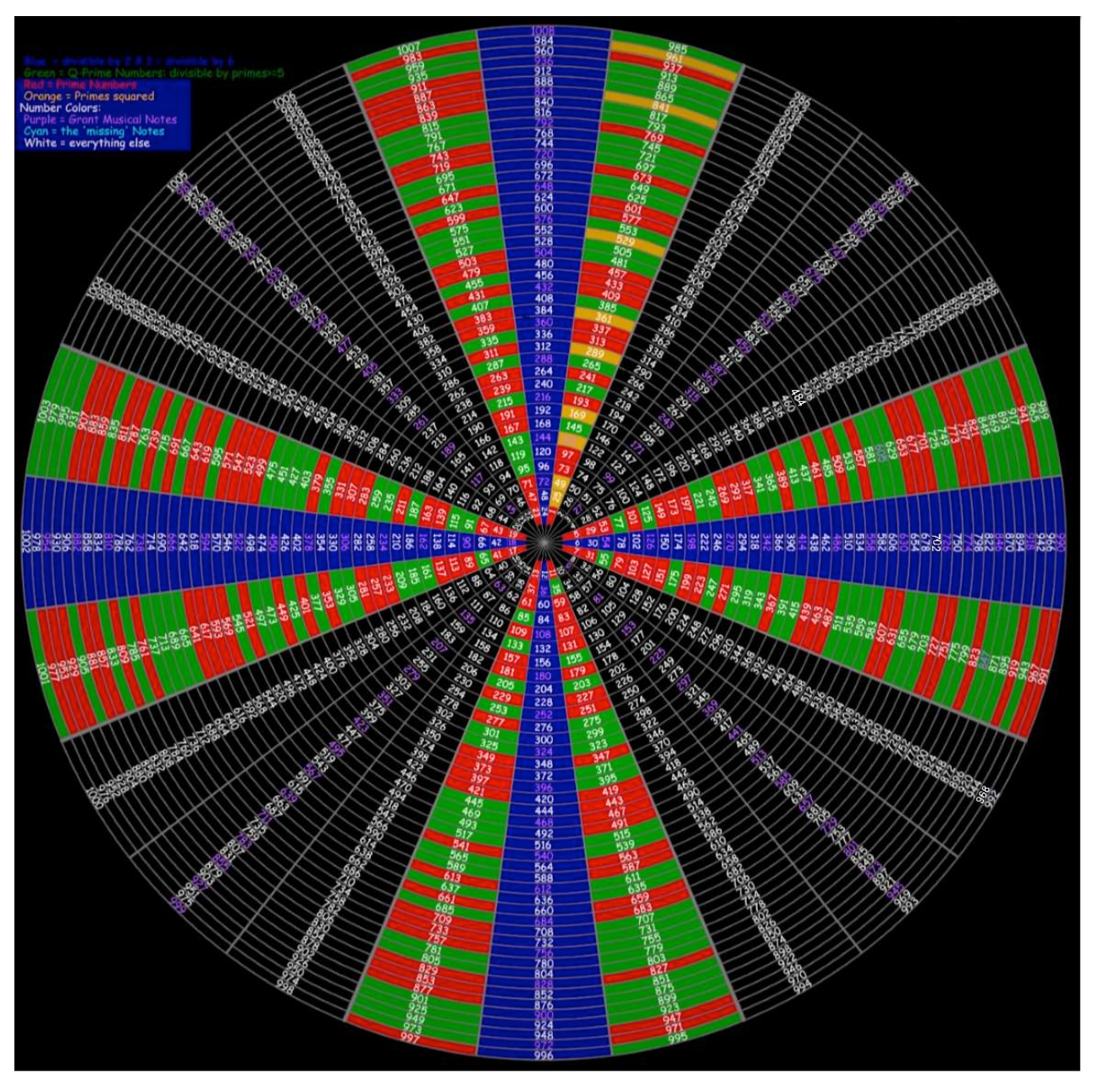
Prime Number Pattern Discovery PUBLISHED
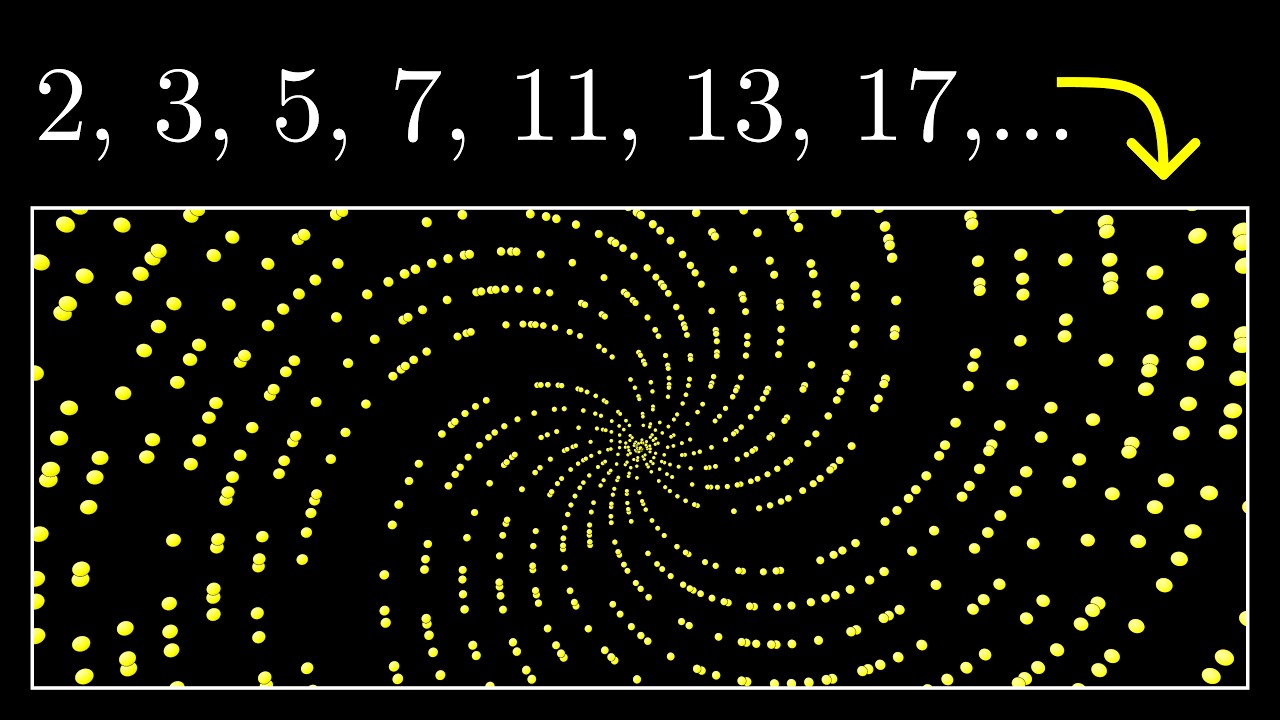
Why do prime numbers make these spirals? Dirichlet’s theorem and pi
For Example, The First 5 Prime Numbers Are 2, 3, 5, 7, And 11.
Web Discover The Fascinating Patterns And Mysteries Of Prime Numbers In This Engaging Video That Explains The Ulam Spiral And Its Implications.
1, 7, 11, 13, 17, 19, 23, 29 1, 7, 11, 13, 17, 19, 23, 29.
I Go Over The Euler Product Formula, The Prime Number Theorem And The Connection Between The Rieman.
Related Post: